Determining the long term solar trend
Posted on 25 March 2008 by John Cook
The most precise measurements of solar activity are satellite observations of Total Solar Irradiance (TSI). TSI is also a useful proxy for other solar activity such as solar flares, cosmic radiation, sunspots, radio flux, UV radiation and x-ray flares - all of which correlate with TSI. However, there is no single continuous record since satellites began taking measurements in 1978. Instead, scientists have had to splice various satellite data together into a single composite record. The two most cited composites are by Frolich and Lean 1998 (PMOD) and Willson 1999 (ACRIM). ACRIM shows a slight increase in solar activity while PMOD shows an even slighter cooling trend.
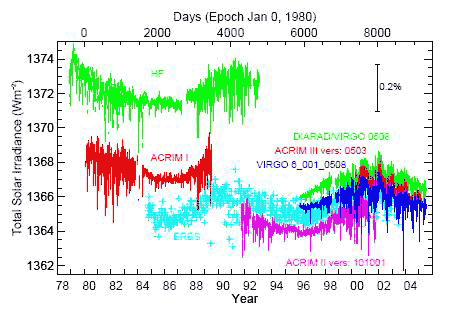
Figure 1: daily averaged values of the TSI from radiometers on different space platforms since November 1978.
Both composites show little long term trend in TSI over the 30 years since satellite measurements began. Scafetta 2006, using the warming ACRIM trend, concludes "since 1975 global warming has occurred much faster than could be reasonably expected from the sun alone." So neither composite indicate the sun has been the primary cause of the last 3 decades of global warming. Nevertheless, determining the more accurate TSI reconstruction is a significant piece in the climate puzzle.
Comparing PMOD to ACRIM
The major difference between the two composites is the handling of data between 1989 and 1991. There is a 2 year gap between ACRIM-I and ACRIM-II (tragically due to the Challenge space shuttle explosion). To fill the gap, both composites use the HF data but in dramatically different ways.
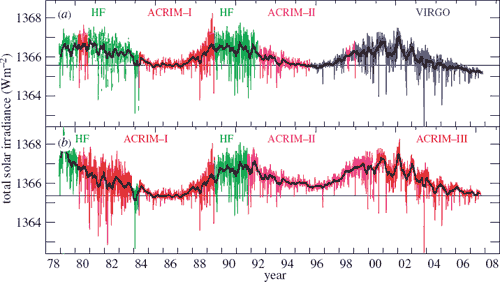
Figure 2: PMOD TSI composite (top) versus the ACRIM TSI composite (bottom). Coloured lines give the daily values with the black solid lines giving the 81 day mean.
PMOD applies corrections to the HF data, which has many sudden jumps due to changes in the orientation of the spacecraft and to switch-offs. Figure 3 demonstrates how the HF corrections are responsible for virtually all of the difference between the long-term drifts of the composites.

Figure 3: The difference between the ACRIM and PMOD composites. The grey line gives the daily values, the black line the 81 day running mean. The step in the ACRIM gap during 1989 is clearly seen and is about half the amplitude of the solar cycle variation.
Another way of comparing the two composites is a scatter plot of TSIACRIM vs TSIPMOD. Most of the data has ACRIM exceeding PMOD - this is for data after 1992. However, there is a small population of points where ACRIM is slightly smaller than PMOD, forming a second, dotted line.
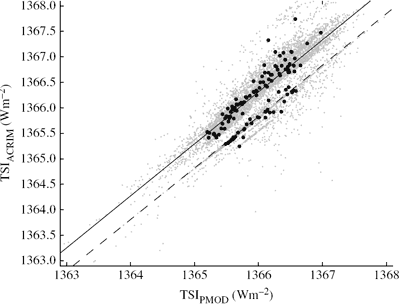
Figure 4: Scatter plot of the daily values (grey) and independent 81-day means (black) of TSIACRIM as a function of the corresponding TSIPMOD value.
Independent tests of the PMOD and ACRIM composites
So which composite correctly handled the HF data? Does TSI dramatically increase during the HF period as ACRIM supposes and the raw HF data indicates? Or did PMOD get their calibrations right when they adjusted the data to show slight solar cooling over the ACRIM gap? Fortunately, there are a number of independent measurements that can confirm solar activity over this period.
- Lee 1995 compares the ERBS satellite data with the Nimbus HF data and found the HF data drifted significantly over the period of the ACRIM gap while the ERBS data shows a slight cooling.
- Krivova 2003 compares TSI to UV levels. UV levels fluctuate more than TSI - a trend would be more visible. As UV correlates closely with TSI, Krivova concludes PMOD is more accurate and there has been little secular trend in TSI over the past few decades.
- A reconstruction of TSI using sunspot numbers (Krivova 2007) found the minimum of cycle 23 was lower than the minimum of cycle 22, in contrast to the ACRIM composite.
- Zurich sunspot counts during the ACRIM gap show a slight downward trend consistent with the PMOD recalibrated data (courtesy of Open Mind).
The latest independent test comes in the form of a new, wordily titled paper, "Recent oppositely directed trends in solar climate forcings and the global mean surface air temperature. II. Different reconstructions of the total solar irradiance variation and dependence on response time scale" (Lockwood 2008). It compares PMOD and ACRIM to a TSI reconstruction based on measurements of solar magnetograms dating back to 1976 (Wenzler 2006). The agreement between PMOD and Wenzler's TSI reconstruction is very good (a correlation coefficient of 0.91). The corresponding correlation with ACRIM is 0.84. Also revealing is a scatter plot of the PMOD composite with Wenzler's reconstruction:
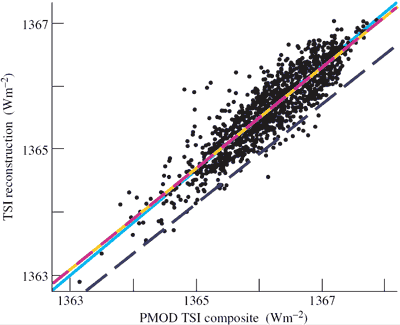
Figure 5: Scatter plot of the daily values of the TSI, as simulated from ground-based magnetograms, as a function of the simultaneous PMOD composite value (1979–2003). The dashed mauve/orange line is the best least-squares linear regression fit and the light blue line is the ideal line of perfect agreement.
If the PMOD composite is correct, the data points should be clustered around the ideal (light blue) line. If ACRIM is correct, a second population should appear aligned along the dashed blue line. Figure 5 shows no such second population. Wenzler's TSI model provides another independent confirmation for the PMOD composite.
So independent tests indicate the PMOD composite is the more accurate TSI reconstruction. The sun has shown a slight cooling trend over the last 3 decades. Not only is the sun not contributing to global warming, it has had a slight, long term cooling effect.































 Arguments
Arguments






























Quite coincidentally, we've been discussing TSI reconstructions although I did this latest PMOD vs ACRIM post because I received a copy of Mike Lockwood's paper last week. But it's neat timing - Krivova's 2007 TSI reconstruction is quite relevant to the PMOD/ACRIM debate.
But please keep the links on topic.