





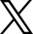




















Latest Posts
Archives
|
 |

Radiative Balance, Feedback, and Runaway Warming
Posted on 26 February 2012 by Chris ColoseSkeptical Science has previously discussed the topic of feedbacks and why the existence of positive feedbacks (i.e., those feedbacks that amplify a forcing) do not necessarily lead to runaway warming, or even to an inherently "unstable" climate system. I also wrote on it at RealClimate (and Pt. 2). This was brought up again in Lord Christopher Monckton's response to SkepticalScience, where he asserted:
"First, precisely because the climate has proven temperature-stable, we may legitimately infer that major amplifications or attenuations caused by feedbacks have simply not been occurring...A climate subject to the very strongly net-positive feedbacks imagined by the IPCC simply would not have remained as stable as it has."
I wanted to revisit the subject in order to take a different approach on the subject of positive feedbacks. This involves the relationship between Earth's surface temperature and outgoing infrared radiation (the energy Earth emits to space). Determining how the outgoing longwave radiation (OLR) depends on surface temperature and greenhouse content is a fundamental determinant to any planetary climate.
I'll begin with very trivial, ideal cases, and then slightly build up in complexity in order to relate the problem to climate sensitivity. By the end, it should be clear why positive feedbacks can exist that inflate climate sensitivity but do not necessarily call for a runaway warming case. We'll also see a scenario, commonly discussed by planetary scientists, in which it does lead to a runaway.
First, we begin with the simplest case in which the Earth has no atmosphere and essentially acts as a perfect radiator. In this case, the outgoing radiation is given by the Stefan-Boltzmann equation OLR=σT4. T is temperature. σ is a constant, so the equation means that the outgoing radiation grows rapidly with temperature, (to the power of four) as shown below.
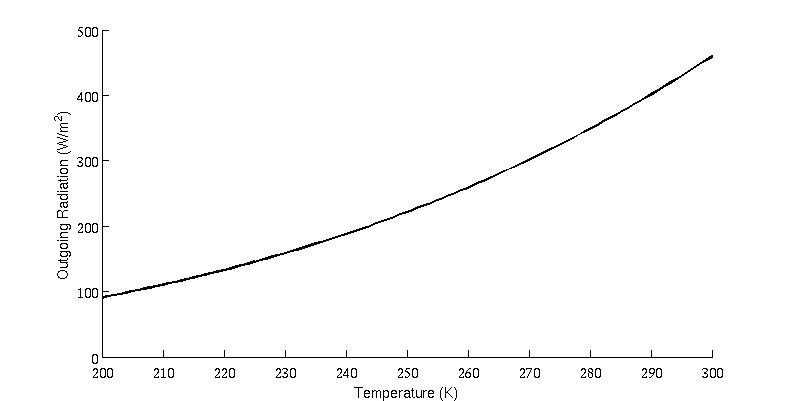
Figure 1: Plot of OLR vs. Surface temperature for a perfect blackbody
In the next case, suppose that we add some CO2 to the atmosphere (400 ppm). The atmosphere here is completely dry (and therefore no water vapor feedback). In this example, the addition of CO2 will reduce the OLR for any given temperature, since the atmosphere absorbs some of the exiting energy. This is displayed with the red curve in Figure 2 (the black curve is from above for reference).

Figure 2: Relationship between OLR and surface temperature for a blackbody (black curve) and with 400 ppm CO2 (red curve). The horizontal line is the absorbed solar radiation.
Also plotted in Figure 2 is a horizontal line at 240 W/m2, which corresponds to the amount of solar energy that Earth absorbs. In equilibrium, the Earth receives as much solar energy as it does emit infrared radiation. Therefore, in the above plot, the points at which the horizontal line intersect the black/red lines will correspond to the equilibrium climates in this model. Note that the red line makes this intersection at a higher surface temperature, which is the greenhouse effect.
Now let's step up the complexity a bit. We'll throw in some water vapor into the model, but not just a fixed amount of water vapor. This time, we'll also let the water vapor concentration increase as temperature increases. Water vapor is a good greenhouse gas, so now the infrared absorption grows with temperature. This is the water vapor feedback. The blue line in the next figure is the OLR for a planet with the same 400 ppm CO2, in addition to this operating feedback.
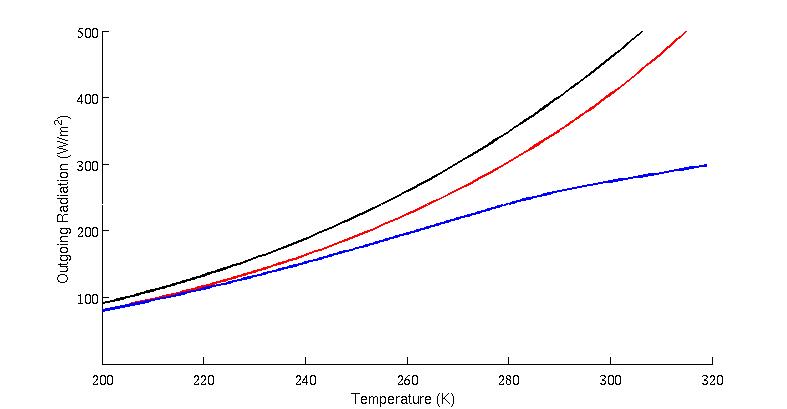
Figure 3: Relationship between OLR and surface temperature, as above, but with a constant relative humidity atmosphere (blue line, implying increasing water vapor with temperature)
In this figure, we see that the OLR does not depend very much on the water vapor at low temperatures. This makes sense, because at temperatures this cold (such as during a snowball Earth), there is so little water vapor in the air. However, at temperatures similar to the modern global mean and warmer, the OLR drops tremendously and the the T4 dependence instead becomes much flatter. We'll get a more clear picture of that means for climate sensitivity in the next diagram.
In the next diagram, I've removed the red curve for convenience. But I've added two horizontal lines this time. You can think of this as two possible values for the incoming solar radiation.

Figure 4: OLR vs. surface temperature for a blackbody (black curve) and an atmosphere with CO2 and a water vapor feedback (blue curve). The horizontal lines give two values for the absorbed incoming solar radiation, and the colored shapes give possible equilibrium points. On the trajectory where water vapor exists, sensitivity is enhanced because the temperature difference between the two red circles (as sunlight goes up) is greater than the difference between the two blue squares.
To interpret Figure 4, suppose that we increase the amount of sunlight that the Earth gets, which means we jump from the red to the green line in the above figure. If the Earth were a blackbody (black curve) then the temperature change that results from this would just be the difference between the values at the two blue squares. However, in a world with a water vapor feedback, the temperature difference is given by the distance between the two red circles. We can infer from this that water vapor has increased climate sensitivity, yet it did not cause a runaway warming effect.
Now let's consider one more case. Notice in the previous diagram that at very high temperatures, the OLR starts to flatten out, and indeed eventually can become almost flat. This is due to the rapid increase in water vapor (and infrared absorption) as temperature goes up. But suppose we pump up the amount of sunlight that the Earth gets to much higher values than in the last figure. This new value is shown by the horizontal green line in the figure below.
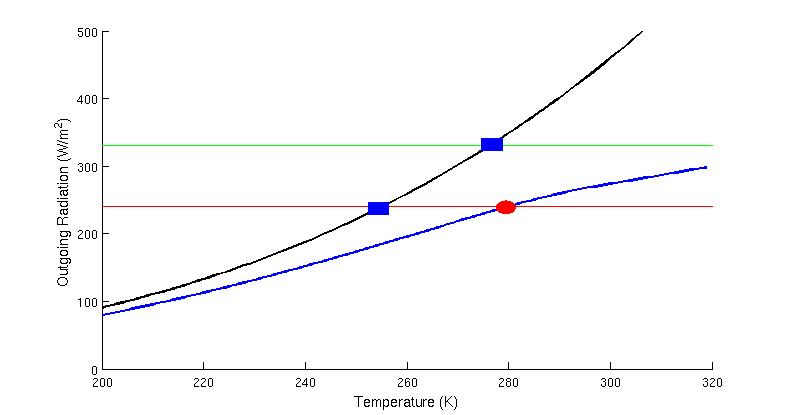
Figure 5: As above, but the green line corresponds to higher incoming solar radiation.
Once again, if we follow the black curve (with no atmosphere), then we get an expected increase in temperature as the amount of sunlight goes up. But if we follow the blue curve (the system with an operating water vapor feedback), then something strange happens.
At some point the OLR becomes so flat, that it can never increase enough to match the incoming sunlight. In this case, it actually becomes impossible to establish a radiative equilibrium scenario, and the result is a runaway greenhouse. This is the same phenomenon planetary scientists talk about in connection with the possible evolution of Venus or exoplanets outside our solar system. The system will only be able to come back to radiative equilibrium once the rapid increase of water vapor mass with temperature ceases, which in extreme cases may not be until the whole ocean is evaporated.
From these figures, we can readily see the fallacy in "positive feedbacks imply instability" type arguments. There is in fact a negative feedback that always tends to win out in the modern climate. This is the increase in planetary radiation emitted to space as temperature goes up. Positive longwave radiation feedbacks only weaken the efficiency at which that restoring effect operates. Instead of the OLR depending on T4, it might depend on T3.9, or maybe even T3 at higher temperatures; eventually the OLR becomes independent of the surface temperature altogether. I haven't discussed shortwave feedbacks, such as the decrease in albedo as sea ice retreats. That only raises the position of the horizontal lines slightly, allowing for a warmer equilibrium point, but in no way compromises the argument.
In fact, the same sort of argument can be applied if we let the albedo vary with temperature (and so the absorbed solar radiation is no longer given by a horizontal line). The opposite extreme, a snowball Earth, can then be thought of as a competition between the decreased longwave radiation to space as the planet cools, and the increased reflection as the planet brightens (when the ice line is advancing toward the equator). As with a runaway greenhouse, it's not inevitable that this occurs, as is evident from times in Earth's history when ice advanced but did not reach the equator.
As a final note, it's worth mentioning that it is virtually impossible to trigger a true runaway greenhouse in the modern day by any practical means, at least in the sense that planetary scientists use the word to describe the loss of any liquid water on a planet. The most realistic fate for Earth entering a runaway is to wait a couple billion years for the sun to increase its brightness enough, such that Earth receives more sunlight than the aforementioned outgoing radiation limit that occurs in moist atmospheres. None of this means that climate sensitivity cannot be relatively high however.
Note: Except for the first graph, all computations here were done using the NCAR CCM radiation module embedded within the Python Interface for Ray Pierrehumbert's supplementary online material to the textbook "Principles of Planetary Climate." The lapse rate feedback is included as an adjustment to the moist adiabat. I've assumed near-saturated conditions are maintained (constant 100% relative humidity) with temperature, although the argument is qualitatively similar with lesser RH values.
This post has been adapted into the Advanced rebuttal to "Positive feedback means runaway warming"
 0 0  0 0
Printable Version | Link to this page
The browser didn't show me #83 until after submitting #84 (in rep to #82).
 0 0  0 0 0 0  0 0 0 0  0 0 0 0  0 0 0 0  0 0 0 0  0 0 0 0  0 0 0 0  0 0 0 0  0 0 0 0  0 0 0 0  0 0 0 0  0 0 0 0  0 0The magnitude and feedbacks of future methane release from the Arctic region are unknown. Despite limited documentation of potential future releases associated with
thawing permafrost and degassing methane hydrates, the large potential for future methane releases calls for improved understanding of the interaction of a changing climate with processes in the Arctic and chemical feedbacks in the atmosphere. Here we apply a “state of the art” atmospheric chemistry transport model to show that large emissions of CH4 would likely have an unexpectedly large impact on the chemical composition of the atmosphere and on radiative forcing (RF). The indirect contribution to RF of additional methane emission is particularly important. It is shown that if global methane emissions were to increase by factors of 2.5 and 5.2 above current emissions, the indirect contributions to RF would be about 250% and 400%, respectively, of the RF that can be attributed to directly emitted methane alone. Assuming several hypothetical scenarios of CH4 release associated with permafrost thaw, shallow marine hydrate degassing, and submarine landslides, we find a strong positive feedback on RF through atmospheric chemistry. In particular, the impact of CH4 is enhanced through increase of its lifetime, and of atmospheric abundances of ozone, stratospheric water vapor, and CO2 as a result of atmospheric chemical processes. Despite uncertainties in emission scenarios, our results provide a better understanding of the feedbacks in the atmospheric chemistry that would amplify climate warming.
Hansen's runaway greenhouse still seems plausible to me. If there had not been past mass extinctions apparently due to massive releases of methane from the hydrates, a true runaway greenhouse would look unlikely.
As it is, with methane release from the hydrates (and probable atmospheric chemistry effects) looking like a plausible explanation for several past mass extinction events, a true runaway greenhouse still looks quite possible to me.
The sun is hotter, now.
Let your modelling approach the complexity of the major feedbacks present in the actual atmosphere of the earth, and I'll admit that you are entitled to make conclusions based on your modelling. At a minimum, you need curves for ozone and nitrous oxide, I think. Keep in mind that the reducing effects of methane on the oceans could plausibly produce large amounts of nitrous oxide.
As it is, you've left out the atmospheric (and oceanic) chemistry effects of methane. The atmospheric chemistry effects of methane are very likely important, and its oceanic chemistry effects may be important.
You could do us all a service by using Isaksen's paper as a guide, and including his stuff in your modelling.
 0 0  0 0Radiative balance is on the title, yet, nothing about it in the post. This is where IPCC is blatantly wrong: on Earth, there has to be radiative imbalance, because there is life.
 0 0  0 0Moderator Response: [DB] Thank you for taking the time to share with us. Skeptical Science is a user forum wherein the science of climate change can be discussed from the standpoint of the science itself. Ideology and politics get checked at the keyboard. When making assertions running counter to accepted science, it is incumbent upon the user (you) to furnish citations to credible sources that support your contentions.
Please take the time to review the Comments Policy and ensure future comments are in full compliance with it. Thanks for your understanding and compliance in this matter.
Prev 1 2
You need to be logged in to post a comment. Login via the left margin or if you're new, register here.
|

|





The Consensus Project Website
THE ESCALATOR

(free to republish)
|




































 Arguments
Arguments
































[DB] Inflammatory snipped.
[DB] Imputations of impropriety and fraud snipped. Please pay more than a passing nod to the Comments Policy of this site.
[DB] "The IPCC models suffer from confirmation bias because they assume that CO2 is responsible for most of the recent warming while ignoring the evidence that 50-80% is attributable to natural causes."
Unsupported assertion. It is incumbent upon you to now provide links to peer-reviewed articles published in reputable journals that document that the evidence you mentions both exists and that the IPCC has ignored it.
You will be held accountable for the above statement.