How do we know more CO2 is causing warming?
What the science says...
| Select a level... |
 Basic
Basic
|
 Intermediate
Intermediate
|
 Advanced
Advanced
| ||||
|
An enhanced greenhouse effect from CO2 has been confirmed by multiple lines of empirical evidence. |
|||||||
Climate Myth...
Increasing CO2 has little to no effect
"While major green house gas H2O substantially warms the Earth, minor green house gases such as CO2 have little effect.... The 6-fold increase in hydrocarbon use since 1940 has had no noticeable effect on atmospheric temperature ... " (Environmental Effects of Increased Atmospheric Carbon Dioxide)
At-a-glance
To make a statement like, "minor greenhouse gases such as CO2 have little effect", is to ignore 160 years of science history. So let's look at who figured out the heat-trapping properties of carbon dioxide and when.
Experiments involving various gas mixtures had demonstrated the heat-trapping properties of water vapour, CO2 and methane in the 1850s. But those effects were yet to be quantified - there were no meaningful numbers. It was to be another 40 years before that happened.
Swedish scientist Svante Arrhenius (1859-1927) was the person who crunched the numbers. The results were presented in a remarkable paper, "On the Influence of Carbonic Acid in the Air upon the Temperature of the Ground", in 1896.
The many calculations in the 1896 paper include estimates of the amounts of CO2 increase or decrease required to drive the climate into a different state. One example used was the Hothouse climate of the Cenozoic, around 50 million years ago. Another was the glaciations of the last few hundred millennia.
To get a temperature rise of 8-9°C in the Arctic, Arrhenius calculated that CO2 levels would have to increase by 2.5 to 3 times 1890s levels. To lower the temperature 4–5°C to return to glacial conditions, he calculated a drop in CO2 was needed of 0.62-0.55 times 1890s levels.
We know CO2 levels in the 1890s from ice-core data. They were around 295 ppm. Let's do the sums. A reduction factor of 0.55 to 0.62 on 295 ppm gives 162.2-183.9 ppm. Modern ice-core measurements representing the past 800,000 years show that in glacial periods, CO2 levels fell to 170-180 ppm.
What we now know due to additional research since 1896 when Arrhenius worked on this, is that CO2 was an essential 'amplifying feedback'. That means changes triggered by long term, cyclic variations in Earth's orbit cause warming or cooling and CO2 release or entrapment in turn. Those changes in CO2 levels affected the strength of Earth's greenhouse effect. Changes in the strength of the greenhouse effect then completed the job of pushing conditions from interglacial to glacial - or vice-versa.
Arrhenius also made an important point regarding water vapour: "From observations made during balloon voyages, we know also that the distribution of the aqueous vapour may be very irregular, and different from the ideal mean distribution." This statement holds true today: water vapour is a greenhouse gas but because water exists in gas, liquid and solid forms in the atmosphere, it is continually cycling in and out of the air. It is distributed in a highly uneven fashion and is uncommon in the upper atmosphere. That's where it differs from CO2.
Once CO2 is up there, it's up there for a long time. As a consequence it has a pretty even distribution: 'well-mixed' is the term. As Arrhenius quantified all that time ago, once it's up there it constantly absorbs and re-radiates heat in all directions. That's why dumping 44 billion tons of it into our atmosphere in just one year (2019 - IPCC Sixth Assessment Report 2022) is a really bad idea.
Please use this form to provide feedback about this new "At a glance" section. Read a more technical version below or dig deeper via the tabs above!
Further details
Good scientific theories are said to have ‘predictive power’. In other words, armed only with a theory, we should be able to make predictions about a subject. If the theory’s any good, the predictions will come true.
Here’s an example: when the Periodic Table of the chemical elements was proposed in 1869, many elements were yet to be discovered. Using the theory behind the Periodic Table, the Russian chemist Dmitri Mendeleev was able to predict the properties of germanium, gallium and scandium prior to their discovery in 1886, 1875 and 1879 respectively. His predictions were found to be correct.
The effect on Earth's greenhouse effect of adding man-made CO2 is predicted in the theory of greenhouse gases. This theory was first proposed by Swedish scientist Svante Arrhenius in 1896, based on earlier work by Fourier, Foote and Tyndall. Many scientists have refined the theory since Arrhenius published his work in 1896. Nearly all have reached the same conclusion: if we increase the amount of greenhouse gases in the atmosphere, the Earth will warm up.
Where there is less agreement is with respect to the exact amount of warming. This issue is called 'climate sensitivity', the amount the temperatures will increase if CO2 is doubled from pre-industrial levels. Climate models have predicted the least temperature rise would be on average 1.65°C (2.97°F) , but upper estimates vary a lot, averaging 5.2°C (9.36°F). Current best estimates are for a rise of around 3°C (5.4°F), with a likely maximum of 4.5°C (8.1°F). A key reason for this range of outcomes is because of the large number of potential climate feedbacks and their variable interactions with one another. Put simply, some are much better understood than others.
What Goes Down…
The greenhouse effect works like this: Energy arrives from the sun in the form of visible light and ultraviolet radiation. The Earth then emits some of this energy as infrared radiation. Greenhouse gases in the atmosphere 'capture' some of this heat, then re-emit it in all directions - including back to the Earth's surface.
Through this process, CO2 and other greenhouse gases keep the Earth’s surface 33°Celsius (59.4°F) warmer than it would be without them. We have added 42% more CO2, and temperatures have gone up. There should be some evidence that links CO2 to the temperature rise.
So far, the average global temperature has gone up by more than 1 degrees C (1.9°F):
"According to an ongoing temperature analysis led by scientists at NASA’s Goddard Institute for Space Studies (GISS), the average global temperature on Earth has increased by at least 1.1° Celsius (1.9° Fahrenheit) since 1880. The majority of the warming has occurred since 1975, at a rate of roughly 0.15 to 0.20°C per decade."
The temperatures are going up, just like the theory predicted. But where’s the connection with CO2, or other greenhouse gases like methane, ozone or nitrous oxide?
The connection can be found in the spectrum of greenhouse radiation. Using high-resolution FTIR spectroscopy, we can measure the exact wavelengths of long-wave (infrared) radiation reaching the ground.
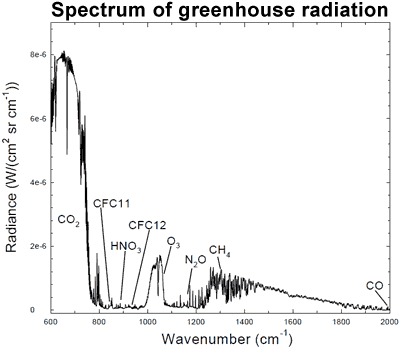
Figure 1: Spectrum of the greenhouse radiation measured at the surface. Greenhouse effect from water vapour is filtered out, showing the contributions of other greenhouse gases (Evans 2006).
Sure enough, we can see that CO2 is adding considerable warming, along with ozone (O3) and methane (CH4). This is called surface radiative forcing, and the measurements are part of the empirical evidence that CO2 is causing the warming.
...Must Go Up
How long has CO2 been contributing to increased warming? According to NASA, “Two-thirds of the warming has occurred since 1975”. Is there a reliable way to identify CO2’s influence on temperatures over that period?
There is: we can measure the wavelengths of long-wave radiation leaving the Earth (upward radiation). Satellites have recorded the Earth's outgoing radiation. We can examine the spectrum of upward long-wave radiation in 1970 and 1997 to see if there are changes.
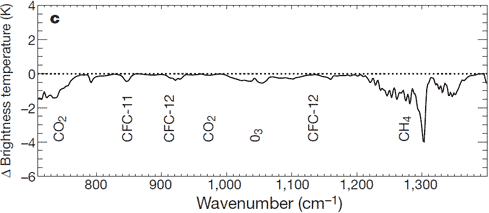
Figure 2: Change in spectrum from 1970 to 1996 due to trace gases. 'Brightness temperature' indicates equivalent blackbody temperature (Harries et al. 2001).
This time, we see that during the period when temperatures increased the most, emissions of upward radiation have decreased through radiative trapping at exactly the same wavenumbers as they increased for downward radiation. The same greenhouse gases are identified: CO2, methane, ozone and so on.
The Empirical Evidence
As temperatures started to rise, scientists became more and more interested in the cause. Many theories were proposed. All save one have fallen by the wayside, discarded for lack of evidence. One theory alone has stood the test of time, strengthened by experiments.
We have known CO2 absorbs and re-emits longwave radiation, since the days of Foote, Tyndall and Arrhenius in the 19th Century. The theory of greenhouse gases predicts that if we increase the proportion of greenhouse gases, more warming will occur.
Scientists have measured the influence of CO2 on both incoming solar energy and outgoing long-wave radiation. Less longwave radiation is escaping to space at the specific wavelengths of greenhouse gases. Increased longwave radiation is measured at the surface of the Earth at the same wavelengths.
Last updated on 16 July 2023 by John Mason. View Archives































 Arguments
Arguments






































Tom Curtis @300. Thank you. Can you tell me if the basic equation is a curve-fitting equation (ie, without the sensitivity factor), or is it derived from fundamental physics? I can't seem to locate something that ought to be related, say, to the partial derivative of enthalpy wrt T at constant p, (∂H/∂T)p. Have you seen something of the sort? My apologies if this is a duplicate. The page did not update with my submission.
[JH] Your prior two duplicate posts have been deleted.
Tom Curtis @300. Thank you. Can you tell me if the formula for radiative forcing is a curve-fitting equation (ie, without the sensitivity factor), or is it derived from fundamental physics? I can't seem to locate something that ought to be related, say, to the partial derivative of enthalpy wrt T at constant p, . Have you seen something of the sort? My apologies if this is a duplicate. The page did not update with my submission, until I took the symbols out.
[JH] They all appeared. Two of the duplicate posts have been deleted.
Tom Curtis @ 300 I've been trying to post a physics question, but the page won't update with my submission, so I'll just use English. Does the forcing equation arise from fundamental physics to your knowledge, or is it something from curve-fitting efforts? Is it possible to put partial differential equations in this text-box?
[JH] All of your prior posts were visible.
Is this post system working? My last three tries have not produced a post after Tom Curtic @ 300.
[JH] Yes, the system is working. All of your comments have appeared.
[RH] What's probably happening is, he's posting at the end of one page and the comments is showing up on the new following page. That one trips people up from time to time.
DrBill@304,
Please show a bit more respect to Mr Curtis by taking care to spell his name correctly. The fact that Mr Curtis in one of the most accomplished commenters on this site calls for even more care.
Then people will show more respect to you and your comments, reciprocally.
chriskoz @305 while I agree that correct spellings of names is a matter of courtesy, DrBill spelled my name correctly in three posts prior to that @304, so it is reasonable to assume that that mispelling was entirely inadvertent. Further, "most accomplished" is a compliment that suggests significant acheivement in a scientific field, whereas I lack even a BSc. I take that as a compliment to my depth of understanding of the topic, for which thankyou. However, while I think is deserved, that depth is limited, especially relative to anybody with a PhD in atmospheric physics.
DrBill @301, the formula for radiative forcing was not directly derived from fundamental physics. Rather, the change in Outgoing Long Wave Radiation at the tropopause, as corrected for radiation from the stratosphere after a stratospheric adjustment (which is technically what the formula determines), was calculated across a wide range of representative conditions for the Earth using a variety of radiation models, for different CO2 concentrations. Ideally, the conditions include calculations for each cell in a 2.5o x 2.5o grid (or equivalent) on an hourly basis, with a representative distribution and type of cloud cover, although a very close approximationg can be made using a restricted number of latituded bands and seasonal conditions. The results are then have a curve fitted to it, which provides the formula. The same thing can be done with less accuracy with Global Circulation Models (ie, climate models).
The basic result was first stated in the IPCC FAR 1990. That the CO2 temperature response (and hence forcing) has followed basically a logarithmic function was determined in 1896 by Arrhenius from empirical data. The current version of the formula (which uses a different constant) was determined by Myhre et al (1998). They showed this graph:
The formula breaks down at very low and very high CO2 concentrations.
Just a quick thanks to Tom CurtiS, JH and RH. FWIW, I tried several ways to make the page show up and not until this evening did I try to quit and relog in, and that seemed to work. I agree with chriskoz about courtesy and thank Tom for recognizing a typo. If JH/RH don't mind, I wouldn't mind seeing my 302,3,4 deleted; 301 had all I had in mind to ask, as it had reference to the partial derivatives that result in Cp. It's late here, for me, and I'll post a new submission tomorrow.
The free energy change of a process is the sum of energy and entropy*temp, and is generally regarded as happening spontaneously when the sum is <0. A version of free energy is that of Helmholtz, whose summation is ΔF (or ΔA) = ΔE -TΔS, but another is the Gibbs ΔG=ΔH-TΔS. The difference between the two expressions is that ΔE and ΔH are not the same thing: ΔE, as I've been used to thinking of it, is now named ΔU, while ΔH = ΔU + Δ(pV). In short, Gibbs Energy includes PV work, while Helmholtz does not when evaluating the possibility of a spontaneous process. A view of this, which I hold, is that the ΔE portion emphasizes the radiative changes, while the ΔH portion includes pV work.
Since the atmosphere not only radiates energy in trying to come to equilibrium, but also does substantial pV work, I believe a model that seems to rely on radiative transfers is not sufficient to explain the climate.
In support of this, I refer the reader to the 1962 and 1976 editions of The U.S. Standard Atmosphere. Google links lead to pdf's and I don't know if anyone wants to wade through dozens of derivations and perhaps 60 pages of notes and explanations, so I won't burden this post with the links themselves, unless someone wants them. In summary, the NASA, NOAA, and a host of other sponsors and contributors determine a model of the atmosphere based on gravity and Cp ((∂H/∂T)p [see @301], and regard CO2, methane and NOx as "trace gases" with no significant impact on temperature. Similarly, the lapse rate -g/Cp suggests the derivation from Gibbs.
The dichotomy is strong enough to get out the old slide rule, imo, and attempt to recognize something fundamental like Cp in the forcing equation. I have not so far.
DrBill @309, radiation models are not climate models. They determine the transfer of radiant energy within the atmosphere given a set of conditions which include well mixed greenhouse gases present, water vapour present, clouds present, and atmospheric density and temperature profiles. They were first developed for the US Airforce as part of its effort to develop infrared guided missiles (Sidewinders), and were later used for the development of FLIR systems, for interpreting microwave radiation from the atmosphere as temperature, and of course, for determining IR radiation from the atmosphere. As such, they can determine the change in Outgoing Infrared Radiation given a change in atmospheric profile, where such changes at the tropopause are also the change in atmospheric forcing.
In 1970, LBL radiation models could produce results as accurate as this if fed detailed atmospheric profile data gathered by a weather balloon:
By 2008 they could produce results with accuracies like this with more approximate profile information:
Further, for determining radiative forcing, the models are generally set with a temperature profile determined by radiative/convective equilibrium in the troposphere (ie, one that follows the adiabatic lapse rate), and with a radiative equilibrium above the tropopause, and therefore complies with the requirements of the laws of thermodynamics (including that for Gibbs free energy).
Of course, radiative models only determine forcing, and not the temperature response to forcing within the troposphere. (They can be used to calculate the initial temperature responce in the stratosphere and above were radiative equilibrium obtains.)
Global Circulation Models, which determine temperature responce as well as radiative response, also include approximations to the laws of thermodynamics governing movement of gases under gravity. (Approximations only given the limits of cell size required to make the models able to operate on modern super computers.)
[JH] Spencer Wart has detailed the history of General Circulation Models here.
Tom Curtis at 11:04 AM on 20 April, 2017
" the formula for radiative forcing was not directly derived from fundamental physics. Rather, the change in Outgoing Long Wave Radiation at the tropopause, as corrected for radiation from the stratosphere after a stratospheric adjustment (which is technically what the formula determines), was calculated across a wide range of representative conditions for the Earth using a variety of radiation models, for different CO2 concentrations."
This seems very odd to me. You use a formula for radiation, not based on fundamental physics?
And the changes in Outgoing Long Wave Radiation is corrected for radiation in the stratosphere, after you adjust the stratosphere in what way?
This is then used to calculate surface temperature, or some influence on it?
Isn´t that an example of doing radiation physics backways? To me it seems like you use the effect as a cause if you start at the last point where radiation leaves the climate system.
Do I understand it right that you adjust stratospheric radiation to co2 concentration, then correct Outgoing Long Wave radiation to that, then use that information as a cause, or part of the cause, of surface temperature?
Is the infrared radiation of the stratosphere the cause of infrared radiation in the tropopause, and the infrared radiation of the tropopause is the cause of infrared radiation from the surface? I see you write radiative forcing, but as I understand it, radiative forcing is still a change of infrared radiation.
Can you connect that to solar radiation? I guess this chain reaction stops at the surface since it is not possible for the surface to have an effect on heat from the sun. I thought a model had to be done in the other way around, you start with the heat source and find the amount of absorbed heat in the surface. Then continue to estimate the heat absorbed by the atmospheres different layers and then it leaves the system. That´s how I learned in school, but that is a long time ago, I guess something has changed.
But I think it is counterproductive to not use fundamental physics to explain temperature, or Outgoing Longwave Radiation. When we learned about solar radiation and the temperature of the atmosphere, our teacher used a basic model for heat. He used the surface temperature and the inverse square law. He said that the solar heat is absorbed and emitted according to the inverse square law and the difference in temperature, or heat flux, is the way to describe earth as a steam engine. That is fundamental physics and it gives the right amount of infrared radiation observed by satellites. Just use the difference and divide whats left by four.
When you say that you use a model doing it backways, in my view, and that it doesn´t use fundamental physics that describe heat, why is your way of doing it better?
I had a look at the link to general circulation models. After reading that my conclusion is that it seems like we are not much wiser
"For all the millions of hours the modelers had devoted to their computations, in the end they could not say exactly how serious future global warming would be. They could only say that it was almost certain to be bad, and unless strong steps were taken soon, it might well be an appalling catastrophe."
This does not convince me that climate models are doing it right by using backwards calculations where emitted radiation is causing the temperature of layers below.
After reading this I don´t get much wiser either:
https://pubs.giss.nasa.gov/docs/2011/2011_Hansen_ha06510a.pdf
It seems like he is saying that if the thermal radiation emitted from the atmosphere, it causes the temperature to increase?
He says that observed heat from the earth is not in balance, the heat flux from the sun that heats earth is larger than the amount of heat that earth emit to space. I find that logical, the earth is not equally warm throughout, and then it has to emit less energy. Only when the system is equally warm in every point inside, it emits as much heat to space as it receives. If we compare to a steam engine and the troposphere is the waterfilled cylinder, then it has to have an even temperature from the surface all the way up to the tropopause before the heat flux is equal to what the sun deliver to the surface.
I really don´t understand this radiative forcing and radiative imbalance, how does it work? How can less heat emitted from the atmosphere cause more heat elsewhere? The more I read about it, the more confused I get.
Hansen wrote about satellite measurements showing an imbalance of 6.5W/m^2 averaged over 5 years. Then he says it was thought to be implausible and they made instrumentation calibrations to align the devices with what the models say, 0.85W/m^2.
He says that the forcings is known accurately, but when measured it is corrected to follow the models. They don´t correct the models according to measurements?
How can forcings be known accurately if they are not a result of measurements? Not any of the studies show how any numbers of forcing has been achieved. And I can´t find any descriptions of the heat flow the way I think it should be done, or rather, the way I like it. Just using measured temperature to find out how much heat there is in the different parts, and from there you can describe the heat flow. That is how we always have done it, and it works. Steam engine, it works perfectly for earth, why use anything else? My teacher must have been very smart, or dumb enough to keep it simple. I was directed here after talking to people in the march and they couldn´t answer my questions. I thought it was strange, so many people marching for science, but no one knew anything about heat, but they all carried signs about temperature increasing and climate problems. I always thought that the issue was about the atmosphere getting so hot that it came near to surface temperature, from top to bottom, because that is what it takes to heat something up usually. But this is just strange and now I think that it must be a good thing Trump won the election. I thought he said some stupid things, but he seems to act like he knows what he´s doing.
I guess I won´t be coming back here anymore. Good Luck with your campaign, you are going to need it.
It was supposed to say
-It seems like he is saying that if the thermal radiation emitted from the atmosphere decrease, it causes the temperature to increase?
vatmark... It's rather interesting that you don't grasp this very simple concept but seem to believe that reflects poorly on the science and Hansen rather than you. It's not uncommon, though. We see a fair number of people who come here in a similar state and post extensive comments on how the entire global scientific community doesn't understands this stuff. People here patiently attempt to explain the science to the best of their abilities, and usually the person questioning the science either storms off, never to be heard from again, or gets so absurdly beligerent that we have to block them from posting.
At some point, in their early education, surely all these folks had to take some science classes. Or, perhaps, many of these people have children who have to take science. If they go through a section and tell the teacher they're wrong, and mark the wrong answers on the tests... They fail the class. Everyone, I believe, generally would find this to be unacceptable. The child needs to learn the materials and understand the current science. And even the child, I would assume, would also understand when they didn't study hard enough or put in enough effort to pass the class.
But somewhere these people grow into adults who, it seems fairly deliberately, choose to not accept the scientific materials, like vatmark here. He's seemingly concluding that the experts in this scientific matter are stating things wrong, even though he doesn't comprehend fundamentally simple science.
So, at some point in their lives, there has to be a transition. A point or a period of time over which they move from understanding they don't understand the science to rejection of the science in order to avoid understanding or cover for the incapacity to understand it.
I just find this whole process perpetually fascinating.
vatmark @312, sorry for my delayed response. I am suffering from poor health at the moment, and am finding it difficult to respond to involved posts in a timely manner. Unfortunately this may mean a further delay in responding to two other posts directed to me by you on another thread, for which I also apologize.
1)
I should hope not, as that is not what General Circulation Models (GCM) do. Rather, they divide the ocean and atmosphere into a number of cells, and for each time step solve for all energy entering, absorbed and emitted from that cell, including energy transfers by radiation, latent heat, diffusion and convection. In doing so, they maintain conservation of energy and momentum (or at least as close an approximation as they can maintain given the cellular rather than continuous structure of the world). When they do this, properties of the simplified models of the greenhouse effect used primarilly for didactic purposes are found to emerge naturally, thereby showing those simplified models to capture essential features of the phenomenon.
2)
You have taken a reqirement for a body, heated externally, and equally from all directions and assumed it is a universal condition. It is not.
To take a simple example, if a spherical body having the same thermal conductivity throughout, bathed in a fluid of uniform temperature, but having a significant heat source at the center. According to you it must have the same temperature throughout before energy in can equal energy out. But, based on Fourier's law of conduction, if there is no temperature gradient, there is no movement of energy by conduction. If follows that based on your theory, the heat from the heat source at the center can never leave, which must result in an infinite energy build up at the center.
Your assumed requirement does not even describe such very simple models. It has been falsified, in fact, since Fourier's experiments that led to his seminal work. It certainly does not apply to the complicated situation of an atmosphere, or a large, massive rotating sphereoid heated intensely from one side, and situated in a heat bath of near zero degrees absolute, ie, to the Earth.
Your claim is also refuted by the Earth itself, which has existed for long enough, with a very stable energy source, that it is in near thermodynamic equilibrium. If your supposed condition held, then there would be no significant difference in temperature with altitude. Despite that, ice has existed at altitude in the tropics for hundreds of thousands of years.
3)
Satellite measurements currently suffer a disadvantage, in that while they are very accurate in showing relative changes in Total Solar Irradiance (TSI) and Outgoing Long Wave Radiation (OLR), they are fairly inaccurate in showing absolute values. This was known from design specifications, and also by comparison of the data from instruments of the same, or different design over the same period, as here:
That means, while we can know the annual change in the energy imbalance quite accurately, we cannot know it's absolute value from satellites alone. Two different methods are used to compensate for this. In the past, the values from climate models were used of necessity. Since the advent of Argos, the rise in OHC is sufficienty well known that it can be used to calibrate the absolute energy imbalance. Hanson discusses both methods (which approximately agree, and certainly agree far better than does either with the value from the satellites). Further, the specific use of computers you mention was not Hanson's, but that of Loeb (2006).
4)
Hanson does not say the forcings are known accurately. Rather, he shows the Probability Density Functions of the forcings:
As can be seen, the 95% confidence limits of the greenhouse gas forcing amount to a range of about 1 W/m^2, or approximately a third of the best estimate forcing. In constrast, the aerosol forcing has a 95% confidence limit range of about 3 W/m^2, or just over twice the best estimate.
5)
Given the level of understanding of thermodynamics shown by you in your claims about equal temperature, it is neither a surprise nor a problem that you cannot find descriptions of heat flow the way you like. GCMs do use, however, the standard laws of thermodynamics, and of heat flow in its various forms.
vatmark @311:
1)
The radiation models used to calculate radiative forcing are based on fundamental physics (ie, basic physical laws). The actual radiative forcing, however, depends on things like extent and type of cloud cover, type of ground cover, surface temperature, etc. These conditions cannot be directly calculated from fuandamental physics, but must be observed. It follows that the radiative forcing also cannot be directly calculated from fundamental physics.
2)
Given a change in atmospheric concentration of a greenhouse gas, or of incoming insolation, the stratosphere will establish effective thermal equilibrium very quickly. As a result it is convenient to define radiative forcing with the stratospheric adjustment. You can do it differently. The Instantaneious Radiative Forcing is calculated without a stratospheric adjustment, for example. However, the values cited in the IPCC are for the adjusted Radiative Forcing.
The stratospheric adjustment would be made by adjusting the stratospheric temperature by successive approximation until energy into the stratosphere equals energy out of the stratosphere, and the various levels of the stratosphere are in local thermodynamic equilibrium. As noted above, I have seen an explicit technique for doing this, but do not currently remember it.
3)
You appear to be confused. A change in radiative forcing can as easilly be due to a change in insolation (your forwards effect) as from a change in green hous gas concentration. The radiative forcing is used because the tropopause is an easilly defined and measured energy boundary. As such, it must satisfy the definition of conservation of energy - ie, that if more energy goes in than out, energy must be stored in some form within the boundary. Given that the energy levels involved are not sufficient for large scale energy to matter conversion, the energy will be stored as heat, and consequently will result in an increase in temperature. It is that fact that makes it possible to calculate the effects of changes in GHG concentration by using the concept of "radiative forcing".
I will note that GCMs and radiative transfer models do not use "radiative forcing" to calculate the consequences of changed GHG concentrations, or solar irradiance. They follow all the energy transfers in a step by step process as described in the preceding post. It is only when we do not have access to GCMs (or in specific contexts radiative transfer models), or we want to calculate approximate results without waiting for the several days or weeks of a GCM run that we make use of radiative forcing.
""Isn´t that an example of doing radiation physics backways?"
I suspect you may be getting confused by the technical definitions here.
The simple definition is:
"The rate of energy change per unit area of the globe as measured at the top of the atmosphere"
It is used as way to put the various sources of radiative change (GHG, aerosols, albedo, change in solar insolation) onto an equal footing (more or less). Dont confuse the way in how the change in a particular forcing is actually measured with how it is recalculated to express it as a change in TOA radiative forcing.
The whole reason is that CO2 is the GHG that we can do something about. Even if not the main cause. The discussion is entertainment.
There’s no such thing as the radiative greenhouse effect. I accept it’s a rather bold statement, so let me explain why;
Do you agree that the heat flow equation (for plane parallel) is: Q = sigma * (T1^4 – T2^4) ?
The net difference between the radiative ENERGY which is emitted from both surfaces will result in HEAT being transferred to the surface of the cooler object and this will result in the TEMPERATURE of the cooler surface increasing. It may also result in the TEMPERATURE of the warmer surface decreasing (if the warmer object does not have it’s own power source). Either way, HEAT will continue to be transferred to the cooler object until thermodynamic equilibrium is achieved, when Q =0. At this point no further HEAT is transferred, although both surfaces continue to emit ENERGY. The TEMPERATURE of both surfaces will thereafter remain constant.
When confronted with this statement of truth, many climate experts will revert to the “restricted emissions” argument where the back radiation from the shell inhibits the warmer object from emitting it’s internal ENERGY thus causing the warmer object to HEAT itself and thus cause an increase in TEMPERATURE of the warmer surface.
If this is your claim, show me the part in the HEAT FLOW equation where it states that the primary object, T1, stops emitting because it is prevented from doing so by the secondary object, T2?
The heat flow equation states that T1 emits fully, at: sigma * T1^4, all of the time; it never has its energy “stopped up” inside of itself. And, the ENERGY from the 2nd body (if it's cooler than the 1st body) can’t act as HEAT. The 2nd body does not stop the 1st one from emitting. The 2nd body never sends back more ENERGY to the first than the first sends to the 2nd, hence the 2nd can never HEAT the 1st.
Photons don’t act like electrons. Photons are bosons. Bosons stack upon themselves and can share the same space. They are waves, not particles. Normal matter is particulate and can NOT stack upon itself…if you try to shove matter together it takes up more space. Much confusion is caused by a misunderstanding between particulate material and photon waves. The waves from the secondary (cooler) object don’t suddenly become part of “the commitment” from the primary (warmer) object…photon waves don’t add up like that. If you take two equal waves and pass them through each other, at some point in the phase overlap the amplitude of the combined wave will double…and also at some point during the overlap the two waves will cancel each other out. But the effect is on the amplitude. The effect is not on the frequency. You would need a change in the frequency in order to increase temperature, but when identical waves combine they do not change their frequency. This is why the waves from the secondary object only resonate and scatter…they can’t do anything to the frequency of the existing vibrations in the primary object.
That’s the plane parallel scenario dealt with.
Now to move to a steel shell around a sphere where the sphere has it’s own internal power source.
If you agree that only net ENERGY can cause HEAT transfer, and providing that you also agree that the only object which experiences HEAT (and thus experiences a TEMPERATURE increase) is that object where, on it’s surface, Powerin > Powerout i.e. if the power received by an object is greater than the power emitted by that object, then the [positive] difference will be manifested as HEAT upon the surface of that object, and this HEAT will increase the TEMPERATURE of that object, then we can proceed as this the essence of the 2nd LoT.
The model that we are now considering is slightly more complicated than the plane parallel model because of the distance between the outside of the sphere (say having radius 1m) and the inside surface of the shell (say having radius 2m): the surface areas of the two objects are not identical. I therefore suggest that it is more convenient to use power densities (with units of W/m2) rather than absolute power values, so we can say HEAT will be transferred across the [single] boundary between the two objects when PowerDensityin > PowerDensityout and that HEAT will be experienced only by that object where the net difference is positive.
When the temperature of the sphere is Tsphere then, at the surface of the sphere, the PowerDensitysphere = sigma * Tsphere4
When the temperature of the shell is Tshell then, at the surface of the shell, the PowerDensityshell = sigma * Tshell4
Let's consider what happens at each surface;
At the surface of the shell, all of the sphere's emissions are received by the shell. However, due the inverse square law, the PowerDensity of the sphere's own emissions are dissipated over the larger area of the shell (and on the dimensions provided we conveniently know that the surface area of the shell is four times that of the sphere). Nevertheless, if at the surface of the shell PowerDensityin > PowerDensityout then the shell will receive HEAT.
At the surface of the sphere, due to the angle of view from the surface of the shell, not all of the shell's emissions are received by the sphere (some will radiate onto another part of the shell's own surface, again without transferring HEAT). Only if Rshell = Rsphere , will 100% of the shell's PowerDensity leaving the inside surface of the shell be received by the surface of the sphere. Nevertheless, if at the surface of the sphere, PowerDensityin > PowerDensityout then the sphere will receive HEAT.
Hopefully, we can still agree on all of the above, because it's still 2nd LoT. If we do agree, then;
If Tsphere is greater (warmer) than Tshell then HEAT may (depending upon the respective PowerDensity values at the inside surface of the shell) be transferred to the shell (and no HEAT will be transferred to the sphere). Conversely, if Tshell is greater (warmer) than Tsphere then HEAT may (depending upon the two respective PowerDensity values at the surface of the sphere) be transferred to the sphere (and no HEAT will be transferred to the shell).
For a sphere with no internal power source of it's own, the concept of introducing a shell around the sphere actually does decrease the rate of cooling experienced by the sphere (than it would otherwise do as is dissipates it's ever decreasing ENERGY into a 0K environment) but unless the shell was warmer than the sphere at the point the shell was introduced, the sphere will never get hotter.
For a sphere with it's own internal power source, the concept of introducing a shell around the sphere does not change the rate at which the sphere dissipates it's ENERGY to satisfy it’s Stefan-Boltzmann commitment i.e. the temperature of the shell does not impair the sphere from radiating it's ENERGY and so the TEMPERATURE of the sphere does not increase (unless the shell was warmer than the sphere at the point it was introduced - but even then, the increase in TEMPERATURE would be transient).
The (false) argument that the introduction of the shell around the sphere prevents the sphere from radiating it's due power density of: sigma * T4 (and so gets warmer from it's own heat) appears to be derived from the erroneous belief that the net energy difference is calculated as the difference between PowerDensityin - minus zero (being the amount it would have received had the shell not been present). This can be shown to be a fallacy if we let the radius of the shell be diminished to it's minimum value i.e. the radius of the shell is reduced to be that of the sphere. In this scenario, the "restricted emissions" argument says that at thermal equilibrium, the PowerDensityshell = PowerDensitysphere and because the shell radiates this on both surfaces, the surface of the sphere also receives PowerDensityshell as HEAT. If this were indeed true, the Sphere would have it's own constant power source of Psphere plus that which receives from the shell (PowerDensityin minus zero), which is Pshell (which is also Psphere) i.e. this is 2*P. But the original model only generates 1 * P. The incorrect "restricted emissions" logic has resulted in the creation of energy (by a factor of two, no less) - something that the 1st LoT says is not possible.
If you now substitute the sphere with internal power source for the Earth and it’s constant ENERGY from our sun and substitute the steel shell for greenhouse gases at the top of the atmosphere then you will now see that the whole radiative Greenhouse effect story has been mathematically busted – the Earth’s surface does not get hotter from back-radiation from the atmosphere.
[PS] This is an absurd travesty of the actual physics. You would possibly benefit from detailed look at the physics at scienceofdoom assuming you want to understand the problem as opposed to trying to convince yourself that you are justified in opposing climate action.
Lampacres @319 , you have tied yourself into a knot, with your "spherical steel shell". To simplify, go back to your "parallel plates" which is a decently fitting approximation of the planetary surface for heat/energy flow per square mile.
Two questions, Lampacres :-
Q1 : How exactly does the high-school physics you mention . . . show any disagreement with mainstream climate science?
Q2 : Have you posted earlier, under the name Cosmoswarrior (etc) ?
[PS] Nice thought but I think it best left to moderators to hunt sockpuppets. Lampacres appears to be someone who stumbled on skydragon nonsense. Hopefully can work through textbook.
Lampacres @ 319:
I will only discuss paragraphs 2 and 3.
Paragraph 2: correct equation for the net exchange between the two plates, but you forgot the other two sides of the plates. What are the inputs/outputs on those sides? Or are you assuming/imposing zero energy flux in any direction other than back and forth between the two plates?
Paragraph 3: you state "...if the warmer object does not have it’s own power source...".
If you are making an analogy with earth, then there is a power source: the sun. It's an impoprtant source, hence my comments about paragraph 2. You're forgetting something.
The other thing you're forgetting, if you are trying to make an analogy with the earth, is that the cooler plate is also losing energy to space. It is also important. Plates have two sides, last I looked.
Try looking over at Eli Rabbet's The Green Plate Effect to see the math done correctly. Also his follow-up post Green Plate Challenge. (If you find yourself agreeing with Betty Pound, then you're in deep physics denial.)
Frankly, I stopped reading closely after you got into the sphere case. You've got the fundamentals of the lane parallel case so wrong that it wasnt worth it. Same mistakes in the sphere section.
[PS] Frankly, I would like Lampacres to answer SoD two questions (and do it on the SoD site) to see whether there is any point to further discussion here. (Another imaginary law of thermodynamics disciples responded to the challenge with this head-vice required answer rather than answering the questions. We dont to go there). The Green plate challenge is another good place to start.
My apologies to Moderators — and to Lampacres, for the insinuation. (The content's validity, and the lengthy style, seemed to bear some similarity.)
The parallel plate analogy needs some improvement. Perhaps try with one plate being semi-transparent. For starters. And possibly the Sun might come into it somewhere, too.
I agree with the statement that CO2 is one of the main driven of climate changes. But it’s not the only one. There are some other things or other gases that can cause climate changes. Actually it’s no matter what can cause climate change more or less we have to prevent it. However, we have to concern on what can cause climate change the most. So, we can find a way to prevent it.
[TD] You are correct that there are other drivers of climate change, but human-caused increase of CO2 is the most important cause of the current warming. Please read the post "CO2 Is the Main Driver of Climate Change."
In Figure 2 comparing spectral absorbtion of outbound radiation 1970 till 1996 why doesn't the O3 absorbtion decrease as the amount of O3 in the atmosphere decreased over that period I expected to see the opposite effect to the CO2 but it seems to be the same effect for a decrease in concentration. I found a chart showing a fall of around 5% O3 over that period:
User 1001,
I could not find a reference that exactly matched your question.
Most (90%) of the atmospheric ozone is in the stratosphere. It blocks incoming UV radiation and protects the Earth's surface. This ozone has degraded in recent decades from attack by chlor-flouro chemicals. The Montreal Protocol has strated the healing process for stratospheric ozone.
At the surface of the Earth (the troposphere) the situation is different. Here ozone is created by pollutants humans release into the atmosphere. The concentration of ozone in the troposphere is increasing. Wikipedia says:
"Quantifying the greenhouse gas potency of ozone is difficult because it is not present in uniform concentrations across the globe. However, the most widely accepted scientific assessments relating to climate change (e.g. the Intergovernmental Panel on Climate Change Third Assessment Report)[47] suggest that the radiative forcing of tropospheric ozone is about 25% that of carbon dioxide."
Ozone in the troposphere absorbs outgoing IR radiation. Thus at the same time as overall concentrations of ozone decreases (causing more UV radiation to reach the surface), there is more ozone in the troposphere causing less UV radiation to get out (the basis of the green house effect). We get screwed both ways.